diff --git a/Grade 10/Math/MPM2DZ/Unit 4: Trigonometry.md b/Grade 10/Math/MPM2DZ/Unit 4: Trigonometry.md
new file mode 100644
index 0000000..825dfc9
--- /dev/null
+++ b/Grade 10/Math/MPM2DZ/Unit 4: Trigonometry.md
@@ -0,0 +1,92 @@
+## Angle Theorems
+
+1. ```Transversal Parallel Line Theorems``` (TPT)
+ a. Alternate Angles are Equal ```(Z-Pattern)```
+ b. Corresponding Angles Equal ```(F-Pattern)```
+ c. Interior Angles add up to 180 ```(C-Pattern)```
+
+ -  +
+2. ```Supplementary Angle Triangle``` (SAT)
+ - When two angles add up to 180 degrees
+
+ -
+
+2. ```Supplementary Angle Triangle``` (SAT)
+ - When two angles add up to 180 degrees
+
+ -  +
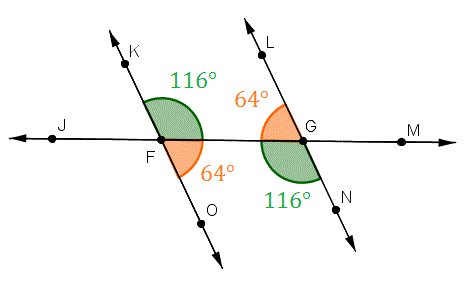
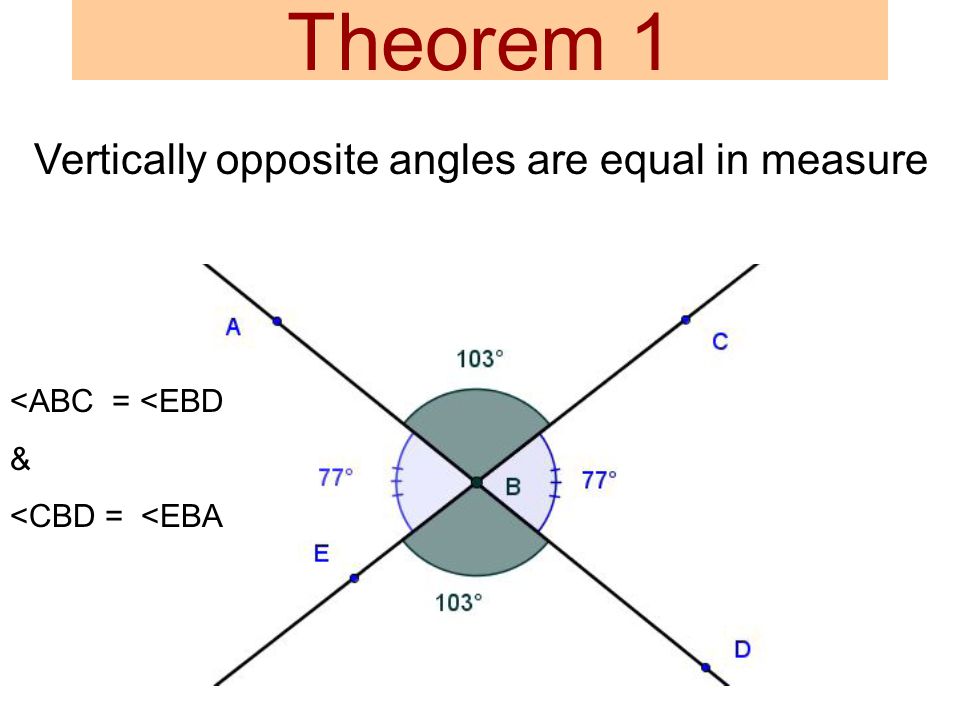
+3. ```Opposite Angle Theorem (OAT)``` (OAT)
+ - Two lines intersect, two angles form opposite. They have equal measures
+
+ -
+
+3. ```Opposite Angle Theorem (OAT)``` (OAT)
+ - Two lines intersect, two angles form opposite. They have equal measures
+
+ -  +
+4. ```Complementary Angle Theorem``` (CAT)
+ - The sum of two angles that add up to 90 degrees
+
+ -
+
+4. ```Complementary Angle Theorem``` (CAT)
+ - The sum of two angles that add up to 90 degrees
+
+ -  +
+5. ```Angle Sum of a Triangle Theorem``` (ASTT)
+ - The sum of the three interior angles of any triangle is 180 degrees
+
+ -
+
+5. ```Angle Sum of a Triangle Theorem``` (ASTT)
+ - The sum of the three interior angles of any triangle is 180 degrees
+
+ -  +
+6. ```Exterior Angle Theorem``` (EAT)
+ - The measure of an exterior angle is equal to the sum of the measures of the opposite interior angles
+
+ -
+
+6. ```Exterior Angle Theorem``` (EAT)
+ - The measure of an exterior angle is equal to the sum of the measures of the opposite interior angles
+
+ - +
+7. ``` Isosceles Triangle Theorem``` (ITT)
+ - The base angles in any isosceles triangle are equal
+
+ -
+
+7. ``` Isosceles Triangle Theorem``` (ITT)
+ - The base angles in any isosceles triangle are equal
+
+ -  +
+8. ```Sum of The Interior Angle of a Polygon```
+ - The sum of the interioir angles of any polygon is ```180(n-2)``` or ```180n - 360```, where ```n``` is the number of sides of the polygon
+
+ -
+
+8. ```Sum of The Interior Angle of a Polygon```
+ - The sum of the interioir angles of any polygon is ```180(n-2)``` or ```180n - 360```, where ```n``` is the number of sides of the polygon
+
+ -  +
+
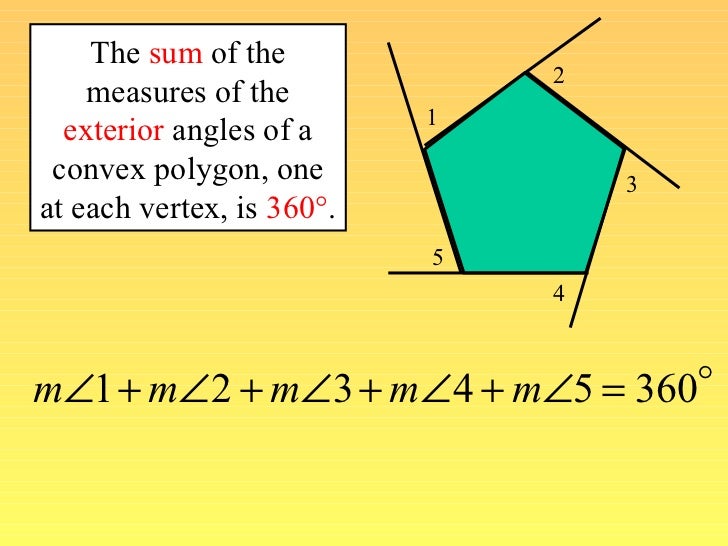
+9. ```Exterior Angles of a Convex Polygon```
+ - The sum of the exterior angle of any convex polygon is always ```360 degrees```
+
+ -
+
+
+9. ```Exterior Angles of a Convex Polygon```
+ - The sum of the exterior angle of any convex polygon is always ```360 degrees```
+
+ -  +
+## Congruency
+`Congruent`: Same size and shape
+
+### Side-Side-Side (SSS)
+
+If three sides of a triangle are respectively equal to the three sides of another triangle, then the triangles are congruent
+
+### Side-Angle-Side (SAS)
+If two sides and the **contained** angle of a triangle are respectively equal to two sides and the **contained** angle of another triangle, then the triangles are congruent.
+
+### Angle-Side-Angle (ASA)
+If two angles and the **contained** side of a triangle are respectively equal to two angles and the **contained** side of another triangle, then the triangles are congruent.
+
+## Similary Triangles
+`Similar`: Same shape but different sizes (one is an enlargement of the other)
+
+### Properties
+
+Lets say we have $`\triangle ABC \sim \triangle DEF`$
+1. Corresponding angles are **equal**
+- $`\angle A = \angle D`$
+- $`\angle B = \angle E`$
+- $`\angle C = \angle F`$
+
+2. Corresponding side are **proportional**.
+- $`\dfrac{AB}{DE} = \dfrac{AC}{DF} = \dfrac{BC}{EF}`$
+
+3. Proportional Area
+- Let $`k`$ be the **scale factor**, when concerning for triangle area, if the triangle area can be defined as $`\dfrac{bh}{2}`$, then by using the smaller triangles side lengths
+our big triangle's area is equal to $`\dfrac{k^2bh}{2}`$. Similar equations and agruments can be dervied from this
+
+### Side-Side-Side similarity (RRR $`\sim`$)
+
+Three pairs of corresponding sides are in the **same ratio**
+
+### Side Angle Side similarity (RAR $`\sim`$)
+Two pairs of corresponding sides are proportional and the **contained** angle are equal.
+
+### Angle-Angle similarity (AA $`\sim`$)
+Two pairs of corresponding angles are equal.
+
+
+
+## Congruency
+`Congruent`: Same size and shape
+
+### Side-Side-Side (SSS)
+
+If three sides of a triangle are respectively equal to the three sides of another triangle, then the triangles are congruent
+
+### Side-Angle-Side (SAS)
+If two sides and the **contained** angle of a triangle are respectively equal to two sides and the **contained** angle of another triangle, then the triangles are congruent.
+
+### Angle-Side-Angle (ASA)
+If two angles and the **contained** side of a triangle are respectively equal to two angles and the **contained** side of another triangle, then the triangles are congruent.
+
+## Similary Triangles
+`Similar`: Same shape but different sizes (one is an enlargement of the other)
+
+### Properties
+
+Lets say we have $`\triangle ABC \sim \triangle DEF`$
+1. Corresponding angles are **equal**
+- $`\angle A = \angle D`$
+- $`\angle B = \angle E`$
+- $`\angle C = \angle F`$
+
+2. Corresponding side are **proportional**.
+- $`\dfrac{AB}{DE} = \dfrac{AC}{DF} = \dfrac{BC}{EF}`$
+
+3. Proportional Area
+- Let $`k`$ be the **scale factor**, when concerning for triangle area, if the triangle area can be defined as $`\dfrac{bh}{2}`$, then by using the smaller triangles side lengths
+our big triangle's area is equal to $`\dfrac{k^2bh}{2}`$. Similar equations and agruments can be dervied from this
+
+### Side-Side-Side similarity (RRR $`\sim`$)
+
+Three pairs of corresponding sides are in the **same ratio**
+
+### Side Angle Side similarity (RAR $`\sim`$)
+Two pairs of corresponding sides are proportional and the **contained** angle are equal.
+
+### Angle-Angle similarity (AA $`\sim`$)
+Two pairs of corresponding angles are equal.
+
+
 +
+2. ```Supplementary Angle Triangle``` (SAT)
+ - When two angles add up to 180 degrees
+
+ -
+
+2. ```Supplementary Angle Triangle``` (SAT)
+ - When two angles add up to 180 degrees
+
+ -  +
+3. ```Opposite Angle Theorem (OAT)``` (OAT)
+ - Two lines intersect, two angles form opposite. They have equal measures
+
+ -
+
+3. ```Opposite Angle Theorem (OAT)``` (OAT)
+ - Two lines intersect, two angles form opposite. They have equal measures
+
+ -  +
+4. ```Complementary Angle Theorem``` (CAT)
+ - The sum of two angles that add up to 90 degrees
+
+ -
+
+4. ```Complementary Angle Theorem``` (CAT)
+ - The sum of two angles that add up to 90 degrees
+
+ -  +
+5. ```Angle Sum of a Triangle Theorem``` (ASTT)
+ - The sum of the three interior angles of any triangle is 180 degrees
+
+ -
+
+5. ```Angle Sum of a Triangle Theorem``` (ASTT)
+ - The sum of the three interior angles of any triangle is 180 degrees
+
+ -  +
+6. ```Exterior Angle Theorem``` (EAT)
+ - The measure of an exterior angle is equal to the sum of the measures of the opposite interior angles
+
+ -
+
+6. ```Exterior Angle Theorem``` (EAT)
+ - The measure of an exterior angle is equal to the sum of the measures of the opposite interior angles
+
+ - +
+7. ``` Isosceles Triangle Theorem``` (ITT)
+ - The base angles in any isosceles triangle are equal
+
+ -
+
+7. ``` Isosceles Triangle Theorem``` (ITT)
+ - The base angles in any isosceles triangle are equal
+
+ -  +
+8. ```Sum of The Interior Angle of a Polygon```
+ - The sum of the interioir angles of any polygon is ```180(n-2)``` or ```180n - 360```, where ```n``` is the number of sides of the polygon
+
+ -
+
+8. ```Sum of The Interior Angle of a Polygon```
+ - The sum of the interioir angles of any polygon is ```180(n-2)``` or ```180n - 360```, where ```n``` is the number of sides of the polygon
+
+ -  +
+
+9. ```Exterior Angles of a Convex Polygon```
+ - The sum of the exterior angle of any convex polygon is always ```360 degrees```
+
+ -
+
+
+9. ```Exterior Angles of a Convex Polygon```
+ - The sum of the exterior angle of any convex polygon is always ```360 degrees```
+
+ -  +
+## Congruency
+`Congruent`: Same size and shape
+
+### Side-Side-Side (SSS)
+
+If three sides of a triangle are respectively equal to the three sides of another triangle, then the triangles are congruent
+
+### Side-Angle-Side (SAS)
+If two sides and the **contained** angle of a triangle are respectively equal to two sides and the **contained** angle of another triangle, then the triangles are congruent.
+
+### Angle-Side-Angle (ASA)
+If two angles and the **contained** side of a triangle are respectively equal to two angles and the **contained** side of another triangle, then the triangles are congruent.
+
+## Similary Triangles
+`Similar`: Same shape but different sizes (one is an enlargement of the other)
+
+### Properties
+
+Lets say we have $`\triangle ABC \sim \triangle DEF`$
+1. Corresponding angles are **equal**
+- $`\angle A = \angle D`$
+- $`\angle B = \angle E`$
+- $`\angle C = \angle F`$
+
+2. Corresponding side are **proportional**.
+- $`\dfrac{AB}{DE} = \dfrac{AC}{DF} = \dfrac{BC}{EF}`$
+
+3. Proportional Area
+- Let $`k`$ be the **scale factor**, when concerning for triangle area, if the triangle area can be defined as $`\dfrac{bh}{2}`$, then by using the smaller triangles side lengths
+our big triangle's area is equal to $`\dfrac{k^2bh}{2}`$. Similar equations and agruments can be dervied from this
+
+### Side-Side-Side similarity (RRR $`\sim`$)
+
+Three pairs of corresponding sides are in the **same ratio**
+
+### Side Angle Side similarity (RAR $`\sim`$)
+Two pairs of corresponding sides are proportional and the **contained** angle are equal.
+
+### Angle-Angle similarity (AA $`\sim`$)
+Two pairs of corresponding angles are equal.
+
+
+
+## Congruency
+`Congruent`: Same size and shape
+
+### Side-Side-Side (SSS)
+
+If three sides of a triangle are respectively equal to the three sides of another triangle, then the triangles are congruent
+
+### Side-Angle-Side (SAS)
+If two sides and the **contained** angle of a triangle are respectively equal to two sides and the **contained** angle of another triangle, then the triangles are congruent.
+
+### Angle-Side-Angle (ASA)
+If two angles and the **contained** side of a triangle are respectively equal to two angles and the **contained** side of another triangle, then the triangles are congruent.
+
+## Similary Triangles
+`Similar`: Same shape but different sizes (one is an enlargement of the other)
+
+### Properties
+
+Lets say we have $`\triangle ABC \sim \triangle DEF`$
+1. Corresponding angles are **equal**
+- $`\angle A = \angle D`$
+- $`\angle B = \angle E`$
+- $`\angle C = \angle F`$
+
+2. Corresponding side are **proportional**.
+- $`\dfrac{AB}{DE} = \dfrac{AC}{DF} = \dfrac{BC}{EF}`$
+
+3. Proportional Area
+- Let $`k`$ be the **scale factor**, when concerning for triangle area, if the triangle area can be defined as $`\dfrac{bh}{2}`$, then by using the smaller triangles side lengths
+our big triangle's area is equal to $`\dfrac{k^2bh}{2}`$. Similar equations and agruments can be dervied from this
+
+### Side-Side-Side similarity (RRR $`\sim`$)
+
+Three pairs of corresponding sides are in the **same ratio**
+
+### Side Angle Side similarity (RAR $`\sim`$)
+Two pairs of corresponding sides are proportional and the **contained** angle are equal.
+
+### Angle-Angle similarity (AA $`\sim`$)
+Two pairs of corresponding angles are equal.
+
+