highschool/Grade 10/Math/MPM2DZ/Unit 4: Trigonometry.md

Unit 4: Trigonometry

Angle Theorems

1. Transversal Parallel Line Theorems (TPT)
   1. Alternate Angles are Equal (Z-Pattern)
      
   2. Corresponding Angles Equal (F-Pattern)
      
   3. Interior Angles add up to 180 (C-Pattern)

• 

2. Supplementary Angle Theorem (SAT)
   

• When two angles add up to 180 degrees

• 

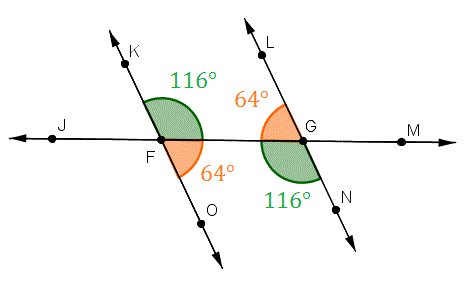
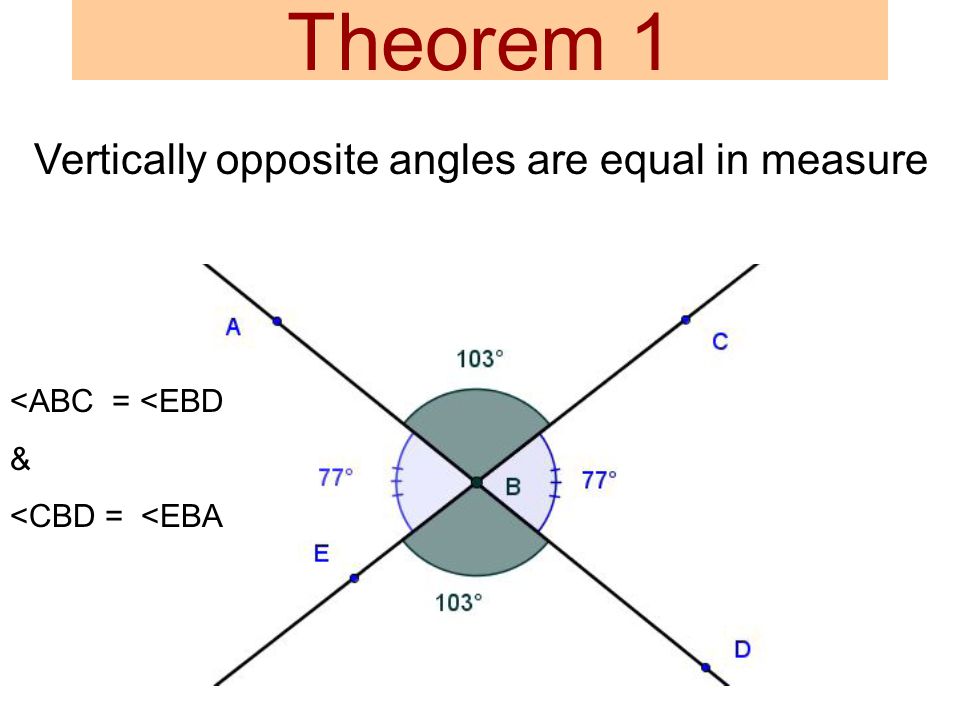
3. Opposite Angle Theorem (OAT) (OAT)
   

• Two lines intersect, two angles form opposite. They have equal measures

• 

4. Complementary Angle Theorem (CAT)
   

• The sum of two angles that add up to 90 degrees

• 

5. Angle Sum of a Triangle Theorem (ASTT)
   

• The sum of the three interior angles of any triangle is 180 degrees

• 

6. Exterior Angle Theorem (EAT)

• The measure of an exterior angle is equal to the sum of the measures of the opposite interior angles

-

7. Isosceles Triangle Theorem (ITT)
   

• The base angles in any isosceles triangle are equal

• 

8. Sum of The Interior Angle of a Polygon

• The sum of the interioir angles of any polygon is 180(n-2) or 180n - 360, where n is the number of sides of the polygon

• 

9. Exterior Angles of a Convex Polygon

• The sum of the exterior angle of any convex polygon is always 360 degrees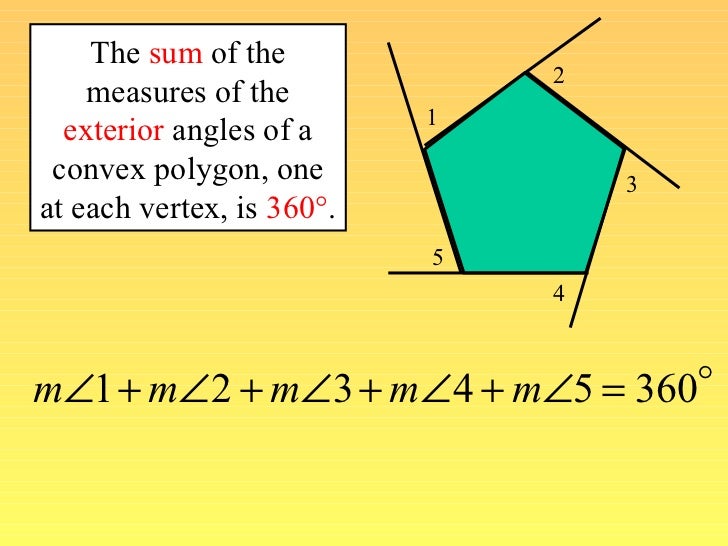

• 

Congruency

Congruent: Same size and shape

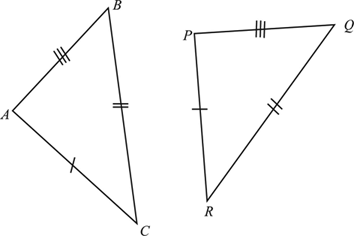
Side-Side-Side (SSS)

If three sides of a triangle are respectively equal to the three sides of another triangle, then the triangles are congruent

Side-Angle-Side (SAS)

If two sides and the contained angle of a triangle are respectively equal to two sides and the contained angle of another triangle, then the triangles are congruent.

Angle-Side-Angle (ASA)

If two angles and the contained side of a triangle are respectively equal to two angles and the contained side of another triangle, then the triangles are congruent.

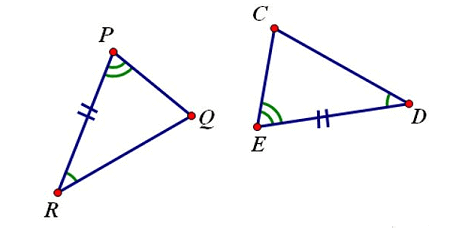
Similar Triangles

Similar: Same shape but different sizes (one is an enlargement of the other)

Properties

Lets say we have \(`\triangle ABC \sim \triangle DEF`\) 1. Corresponding angles are equal - \(`\angle A = \angle D`\) - \(`\angle B = \angle E`\) - \(`\angle C = \angle F`\)

2. Corresponding side are proportional.

• \(`\dfrac{AB}{DE} = \dfrac{AC}{DF} = \dfrac{BC}{EF}`\)

3. Proportional Area

• Let \(`k`\) be the scale factor, when concerning for triangle area, if the triangle area can be defined as \(`\dfrac{bh}{2}`\), then by using the smaller triangles side lengths our big triangle’s area is equal to \(`\dfrac{k^2bh}{2}`\). Similar equations and agruments can be dervied from this

Side-Side-Side similarity (RRR \(`\sim`\))

Three pairs of corresponding sides are in the same ratio

Side-Angle-Side similarity (RAR \(`\sim`\))

Two pairs of corresponding sides are proportional and the contained angle are equal.

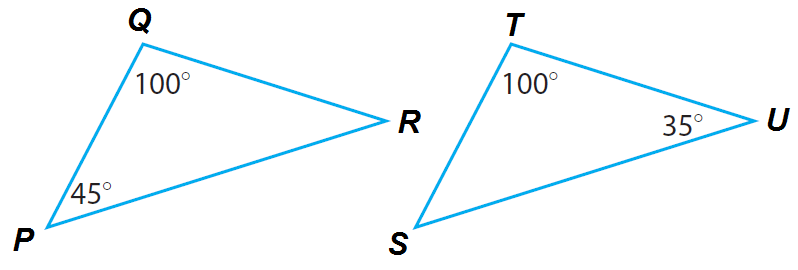
Angle-Angle similarity (AA \(`\sim`\))

Two pairs of corresponding angles are equal. In the diagram below, we can solve for the missing angle using Angle Sum Of A Triangle Theorem (ASTT) and see that those 2 triangle’s angles are equal.

Primary Trigonometric Ratios

Part Of Triangle	Property
Hypotenuse	The longest side of the right triangle. it is across the \(`90^o`\) (right angle)
Opposite	The side opposite to the reference angle
Adjacent	The side next to the reference agnle

Remember: Primary trigonometric ratios are only used to find the acute angles or sides of a right-angled triangle

SOH CAH TOA

SINE \(`\sin \theta = \dfrac{\text{Opposite}}{\text{Hypotenuse}}`\)

COSINE \(`\cos \theta = \dfrac{\text{Adajacent}}{\text{Hypotenuse}}`\)

TANGENT \(`\tan \theta = \dfrac{\text{Opposite}}{\text{Adajacent}}`\)

Angle Of Elevation And Depression

	Angle of Elevation	Angle of Depression
Definition	Angle of Elevation is the angle from the horizontal looking up to some object	Angle of Depression is the angle frorm the horizontal looking down to some object
Diagram

We can see that Angle of Elevation = Angle of Depression in the diagram below (Proven using Z-pattern)

Sine Law

In any \(`\triangle ABC`\): \(`\dfrac{\sin A}{a} = \dfrac{\sin B}{b} = \dfrac{\sin C}{c}`\) or \(`\dfrac{a}{\sin A} = \dfrac{b}{\sin B} = \dfrac{c}{\sin C}`\)

We can derive the formula further to get: - \(`\dfrac{\sin A}{\sin B} = \dfrac{a}{b}`\) - \(`\dfrac{\sin A}{\sin C} = \dfrac{a}{c}`\) - \(`\dfrac{\sin B}{\sin C} = \dfrac{b}{c}`\)

Also, for some trigonometry identities: - \(`\tan x = \dfrac{\sin x}{\cos x}`\) - \(`\sin^2 A + \cos^2 A = 1`\)

If you are finding the sides or agnles of an oblique triangle given 1 side, its opposite angle and one other side or angle, use the sine law.

Ambiguous Case

The ambiguous case arises in the SSA or (ASS) case of an triangle, when you are given angle-side-side. The sine law calculation may need to give 0, 1, or 2 solutions.

In the ambiguous case, if \(`\angle A, a, b`\) are given, the height of the triangle is \(`h= b\sin A`\)

Case	If \(`\angle A`\) is acute	Condition	# & Type of triangles possible
1		\(`a \lt h`\)	no triangle exists
2		\(`a = h`\)	one triangle exists
3		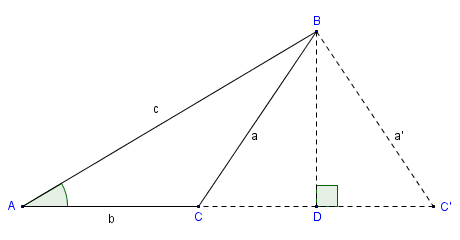\(`h \lt a \lt b`\)	two triangle exist (one acute triangle, one obtuse triangle)
4		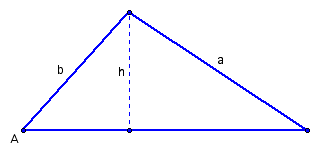\(`a \ge b`\)	one triangle exists

Case	If \(`\angle A`\) is obtuse	Condition	# & Type of triangles possible
5		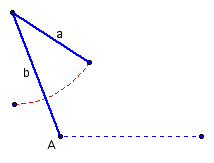\(`a \le b`\)	no triangles exist
6		\(`a \gt b`\)	one triangle exists

Cosine Law

In any \(`\triangle ABC`\), \(`c^2 = a^2 + b^2 - 2ab\cose C`\)

If you are given 3 sides or 2 sides and the contained angle of an oblique triangle, then use the consine law

Directions

Bearings: Always start from North, and goes clockwise Direction: Start from the first letter (N, E, S, W), and go that many degrees directly to the second letter (N, E, S, W)

Note: Northeast, southeast, northwest etc. all have 45 degrees to the left or the right from their starting degree (0, 90, 180, 270)

2D Problems

Note: Watch out for the case where you don’t know which side the 2 things (buildings, boats, etc.) are, they can result in 2 answers

3D problems

Note: Use angle theorems to find bearing/direction angle, and to help with the problem in general. Apply sine law, cosine law, and primary trigonometric ratios whenever necessary.