highschool/Grade 9/Math/MPM1DZ/Unit 6: System of Equations.md

Unit 6: System of Equations

Linear System

• Two or more equation that you are working on all together at once on the same set of axes.
  

• The lines may cross or intersect at a point called the Point of Intersection (POI).
  

• The coordinated of the POI must satisfy the equation of all the lines in a linear equation.

• In business, the Point of Intersection is known as the Break Even Point where Revenue - Cost = Profit
  

• when Profit = 0. There is no gain or loss.

Number of Solutions

• 

Discriminant

• The discriminant determines the number of solutions (roots) there are in a quadratic equation. \(`a, b , c`\) are the

• coefficients and constant of a quadratic equation: \(`y = ax^2 + bx + c`\)
  \(` D = b^2 - 4ac \begin{cases} \text{2 distinct real solutions}, & \text{if } D > 0 \\ \text{1 real solution}, & \text{if } D = 0 \\ \text{no real solutions}, & \text{if } D < 0 \end{cases} `\)

• 

Solving Linear-Quadratic Systems

• To find the point of intersection, do the following:

  1. Isolate both equations for \(`y`\)
  2. Set the equations equal to each other by subsitution Equation 1 = Equation 2
  3. Simplify and put everything on one side and equal to zero on the other side
  4. Factor
  5. Use zero-product property to solve for all possible x-values
  6. Subsitute the x-values to one of the original equations to solve for all y-values
  7. State a conclusion / the solution

• 

• There are 3 possible cases
  

• In addition, to determine the number of solutions, you the Discriminant formula \(`D = b^2 - 4ac`\)

Ways to solve Systems of Equations

## 1. Subsitution
- Here we eliminate a variable by subbing in another variable from another equation
- We usually do this method if a variable is easily isolated
- Example: - y = x + 10 (1) x + y + 34 = 40 (2) - We can sub \(`(1)`\) into \(`(2)`\) to find \(`x`\), then you the value of \(`x`\) we found to solve for \(`y`\) x + (x + 10) + 34 = 40 2x + 44 = 40 2x = -4 x = -2 - Then solve for \(`y`\)
y = -2 + 10 y = -8

## 2. Elimination - Here we eliminate a variable by basically eliminate a variable from an equation
- We usually use this method first when the variables are not easily isolated, then use subsitution to solve
- Example: - 2x + 3y = 10 (1) 4x + 3y = 14 (2) - We can then use elimination 4x + 3y = 14 2x + 3y = 10 ------------ 2x + 0 = 4 x = 2 - Then sub the value of \(`x`\) into an original equation and solve for \(`y`\)
2(2) + 3y = 10 3y = 6 y = 2

3. Graphing

• we can rewrite the equations into y-intercept form and then graph the lines, and see where the lines intersect (P.O.I), and the P.O.I is the solution

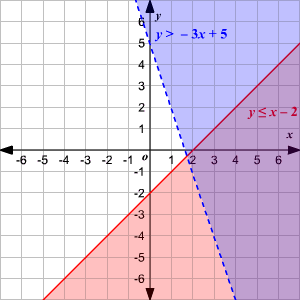
Solving Systems of Linear Inequalities

• Find the intersection region as the solution.
  

• If

• 	Use Dash line	Use Solid line
  Shade the region above the line	\(`y > mx + b`\)	\(`y \ge mx + b`\)
  Shade the region below the line	\(`y < mx + b`\)	\(`y \le mx + b`\)

• If

  • \(`x > a`\) \(`x \ge a`\)
    shade the region on the right

• If

  • \(`x < a`\) \(`x \le a`\)
    shade the region on the left

• Step 1. change all inequalities to y-intercept form
  

• Step 2. graph the line
  

• Step 3. shade the region where all the regions overlap

• 

Tips

• Read the questions carefully and model the system of equations correctly
  
• Be sure to name your equations
  
• Label your lines